Oscillating Systems

Calculate the time period of a simple pendulum of length $1.44\mathrm{m}$ on the surface of the moon. The acceleration due to gravity on the surface of the moon is$\raisebox{1ex}{$1$}\!\left/ \!\raisebox{-1ex}{$6$}\right.$ the acceleration due to gravity on earth.{$g=9.8 {\mathrm{ms}}^{-2}$ }

A pendulum clock gives correct time at $20°C$ and at a place where $g=9.8 m/s^2$.It is taken to a hill where the acceleration of gravity is
 $g=9.788 m/s^2.$ At what temperature will it give correct time? Coefficient of linear expansion of steel =$12\times {10}^{-1}{{}^{0}C}^{-1}.$

How much time a clock will gain or lose when taken from a place at $20° C$ to a place having the temperature $40°\mathrm{C}$?

. The coefficient of cubical expansion of iron=$36\times {10}^{-6 }°{\mathrm{C}}^{-1}$

A mass $\mathrm{M}$ is suspended with a weightless spring. When the spring is slightly pulled and released then the mass oscillates with the time period $\mathrm{T}$. If the mass is increased by $\mathrm{m}$, then the time period becomes $\frac{5\mathrm{T}}{3}$. Determine the ratio $\frac{\mathrm{m}}{\mathrm{M}}$.

A mass $\mathrm{m}$ is suspended one by one by two springs of force constants ${\mathrm{k}}_1, {\mathrm{k}}_2$. The time periods of their oscillations are ${\mathrm{T}}_1 \mathrm{and} {\mathrm{T}}_2$ respectively. If the same mass be suspended by connecting the two springs in parallel then the time period of oscillations is $\mathrm{T}$.The correct relation is:

Derive an expression for the time period of two loaded springs in parallel combination.

Find an expression for the time period of a vertical spring-mass system.

What will be the effect on the time period  $\mathrm{T}$of a pendulum using a copper wire, if it has been taken to a room where the temperature is higher by $30°C$.

Discuss the effect of temperature on the time period of simple pendulum.

What is percentage change in the time period, if the length of the simple pendulum increases by $3\%$?

A pendulum clock at the hills  will:

According to the law of the simple pendulum $\mathrm{T}\propto \sqrt{\mathrm{l}}$. Does it mean that when $l=\infty , T=\infty$? explain.

Mention the laws of simple pendulum.

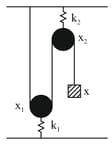
The time period for small vertical oscillations of a block of mass $\mathrm{m}$ when the masses of the pulleys are negligible and spring constant ${\mathrm{k}}_1$ and ${\mathrm{k}}_2$ is

A light spring of force constant $8 {\mathrm{Nm}}^{-1}$ is cut into two equal halves and the two are connected in parallel; the equivalent force constant of the system is

A light spring of constant $k$ is cut into two equal parts. The spring constant of each part is

In a spring-mass system, the length of the spring is $L$, and it has a mass $M$ attached to it and oscillates with an angular frequency $\omega$. The spring is then cut into two parts, one (a) with relaxed length $\alpha L$and the other (b) with relaxed length $\left(1-\alpha \right)L$. The force constants of the two springs $A$ and $B$ are

In case of a simple pendulum, time period versus length is depicted by

A simple pendulum of length $1 \mathrm{m}$ has mass $10 \mathrm{g}$ and oscillates freely with amplitude of $5 \mathrm{cm}$. Its potential energy at extreme position is

A simple pendulum with a bob of mass $40 \mathrm{g}$ and charge $+2 \mathrm{\mu C}$ makes $20$ oscillations in $44$ seconds. A vertical electric field of magnitude $4.2\times {10}^4 {\mathrm{NC}}^{-1}$ pointing downward is applied. The time taken by the pendulum to make $15$ oscillations in the electric field is (Acceleration due to gravity $=10 {\mathrm{ms}}^{-2}$)